Solution: We begin our analysis by first drawing the free-body diagram of the structure. Once we have the unknown reaction loads identified, we solve for them using the equilibrium equations.

Free-Body Diagram: The structure is fixed at point A and pinned at point E. In addition, member BDE is resting on beam ABC through a roller at point B. Let's draw the free-body diagram of the structure as a whole first.

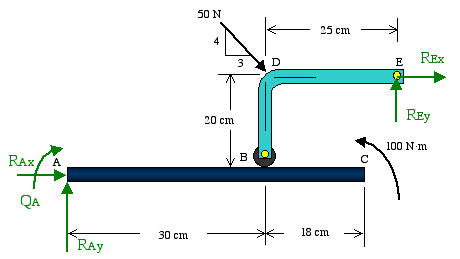
Notice that we are dealing with a two-dimensional force system, which means we can only use three equations of equilibrium. When we look at the free-body diagram above, we see five unknown reactions. So what should we do?

If member BDE were an inseparable part of the structure, we could not solve for the reactions using the equations of equilibrium alone. However, in this case there is a single roller at point B. From our previous discussion on support reactions we know that in such a case, there is only one unknown force in the normal direction. Recognizing this fact alone is the key in being able to solve for the reactions in this problem.

Since we know that if a structure is in the state of equilibrium, every part of it is also in equilibrium, we can split the structure into two separate members as shown below.

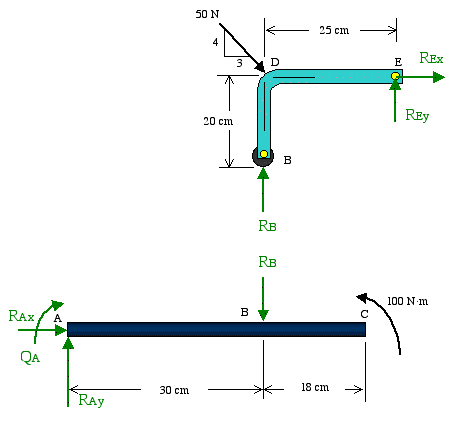
We cannot start the analysis using the free-body diagram of the beam ABC as it contains 4 unknown loads. So we begin with member BDE as it has only three unknown forces. A closer look at member BDE reveals that it is in fact a three-force member. How do we know that? Well, BDE is under the action of one force at B, one force at D, and one force at E, which is the resultant of REx and REy. To satisfy force and moment equilibrium, the three forces must be concurrent. In this case the point of concurrency is point D. This means that the y component of the force at E (i.e., REy) is in fact zero. Although this recognition is helpful in getting to the final answer more efficiently, it is not essential in the analysis of unknown forces. This is because the free-body diagram of BDE involves only three unknown forces, so we can solve for them using the three equilibrium equations.

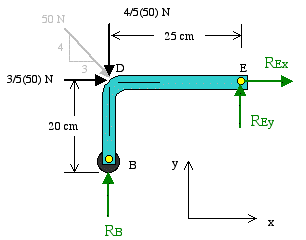
Let's start the solution by using the equilibrium of moments with point E as the moment center and counter clockwise direction as positive. We also split the 50 N force into two components along x,y axes.

=>

=>

Positive sign confirming the assumed direction is correct. Now by summing forces in the x and y directions we solve for the unknown reactions at point E.

=>

=>

Negative sign indicates the direction is opposite to that shown in the free-body diagram. Hence, REx is pointing to the left instead of right.

=>

=>

Verification of Results: Recall that member BDE was recognized as being a three-force member. With the forces acting on it not parallel, they must be concurrent. We can see this in the figure below.

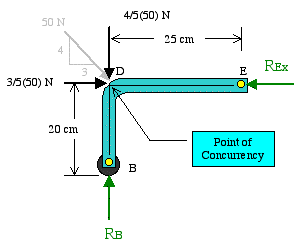
Therefore, point D is the point of concurrency in this case.

Having determined the force at point B, we can now use the free-body diagram of beam ABC to solve for the unknown reaction loads at A.

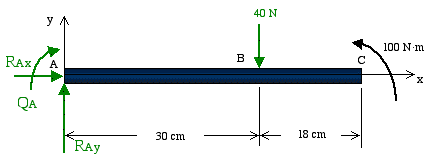
We begin by using the equilibrium of moments with point A as the moment center. We must be careful with the units because of the presence of the couple moment at C with units of N·m.

=>

=>

Positive sign confirming the assumed direction is correct. Now by summing forces in the x and y directions we solve for the unknown reaction forces at point A.

=>

With no other force in the x direction, RAx is zero.

=>

=>

Verification of Results: We confirm the solution by checking the summation of moments about point C to see if it is equal to zero.

=>

=>

With e being zero, we have confidence that there is no error in the solution.