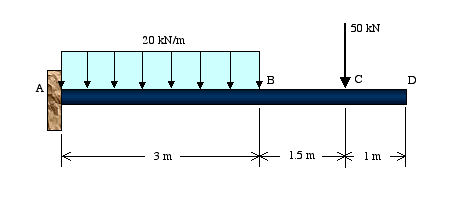
Example 6: For the cantilever beam and loading shown, determine the reactions at the support.
|
Solution: We begin our analysis by first drawing the free-body diagram of the beam. Once we have the unknown reaction loads identified, we solve for them using the equilibrium equations.
Free-Body Diagram of Beam: The beam is fixed at point A. Therefore, there are two reaction forces and one reaction moment at this point as shown below.
We assume a direction for each reaction load. Also to simplify the calculations, the distributed force is represented by its resultant acting at its centroid.,

Reaction loads: As shown in the free-body diagram, there are three unknown reactions that need to be solved for using the equilibrium condition. Since this represents a two-dimensional force system, we can only make use of three equilibrium equations.
We begin the solution by using the equilibrium of moments with point A as the moment center. We choose point A as it would eliminate the contributions of the two unknown reaction forces.
| => |
| => |
Negative sign indicates the direction of QA is opposite to that shown in the free-body diagram. Now we proceed with solving the two reaction forces. Using the equilibrium of forces in the x direction gives
| => |
The horizontal reaction force at A is zero as there is no other horizontal force acting on the beam.
| => |
| => |
Verification of Results: We can verify the solution by summing moments about D or any other point to see if it is equal to zero.
| => |
| => |
With e being zero, we have confidence that there are no errors in the solution.
Unlike in examples 4 and 5, the entire load is supported at point A. The fixed support also develops a moment reaction as the beam is restrained from rotation. This element is an important factor in design of beams.