Moment about an Axis: Consider the
force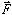 
passing
through point A as shown in the figure.
The view from the y axis reveals that 
where  |
|
where  is the moment
arm of the force with respect to the y axis. In this case, the
moment axis is pointing in the negative y direction. Also as to be
expected,  since 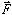is parallel to the z
axis.
|
is the moment
arm of the force with respect to the x axis. In this case, the
moment axis is pointing in the positive x direction as shown.
Similarly, the We can now expand this discussion to the case of calculating the moment about an arbitrary line aa. Two commonly encountered cases are described next.
| Case 1: The line of action of the force
is perpendicular to aa, and that the two
lines do not intersect each other.
In this case, the moment about aa is found as
An example of this case is shown in the figure where the line of action of the force is in z direction and line aa is in xy plane; clearly, they are perpendicular to each other. |
|
|
Case 2: The line of action of the force
is NOT perpendicular to aa, and that the
two lines do not intersect each other.
In this case, the moment about aa is found in two steps using the vector approach. First, the moment about a point lying on line aa is calculated as
Then, the projection of
|
|
|
The previous two equations can be combined into a triple scalar
product as
|
|
If 
comes out negative, it simply means that its direction is opposite
to that defined by  This projection can also be put in vector form as
|
(6)
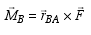
along line 
(Magnitude of the component)
(7)
(8)