Example 4: The beam shown below is under the action of three forces and a couple moment. Determine the magnitude, direction, and location of the resultant force.

Solution (Scalar Approach): We sum forces in the x and y directions to find the two components of the resultant force
| => |
| => |
Hence, the magnitude and direction of the resultant force are found as
![]()
![]()
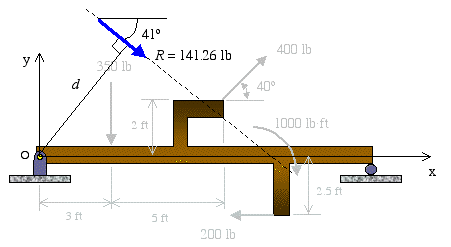
Now by summing moments about point O we will find the line of action (or axis) of the resultant force. We are assuming that the resultant force is to the right of point O as shown above.
Since d, is found to be positive, the assumed position for the resultant axis is correct.
Recall from the principle of transmissibility that a vector can slide anywhere along its axis without changing its effect. Therefore, to find the intersection of the force vector and the beam, we can slide the force down to the x axis, split it into its x,y components, and sum moments about point O in order to solve for distance dx,.
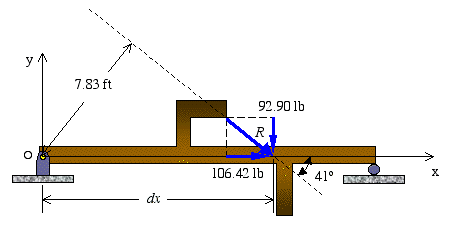
Since the moment of the resultant is equal to the sum of the moments of its components, we can write
Having found the value of dx, we know the location of the resultant force on the beam.